Biot-Savart Law-

 

Before you go through this article, make sure that you have gone through the previous article on Biot-Savart Law.

 

We have learnt-

• In 1820, Oersted discovered that a current carrying conductor produces a magnetic field around it.
• Biot-Savart law helps to calculate the magnetic field due to the current element of a thin wire.
• The magnetic field at any point due to the current element is given by-

 

 

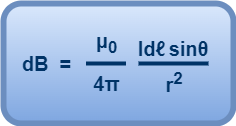
In this article, we will learn about the magnetic field on the axis of a circular loop.

 

Magnetic Field On Axis of Circular Current Carrying Loop-

 

Consider a circular loop of wire of radius R carrying current I and placed with its plane perpendicular to the plane of paper.

 

We wish to determine the magnetic field at an axial point P located at a distance x from the center O of the loop.

 

The entire loop can be assumed to be made up of a large number of small current elements. We consider one such current element at the top of the loop as shown-

 

 

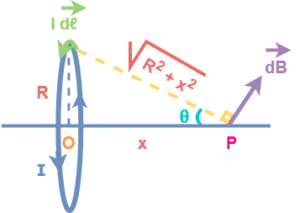
Using Biot-Savart law, the magnitude of the magnetic field dB at point P due to this current element is given by-

 

 

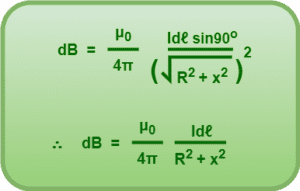
The magnetic field dB can be resolved into two components-

• dBsinθ along the axis
• dBcosθ perpendicular to the axis

 

 

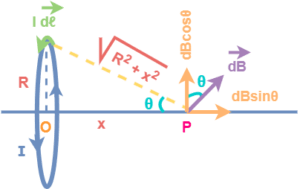
For any two diametrically opposite elements of the loop,

• the components perpendicular to the axis of the loop will be equal and opposite and will cancel out.
• their axial components will be in the same direction and get added up.

 

This is shown in the following figure by considering  diametrically opposite element at the bottom of the loop-

 

 

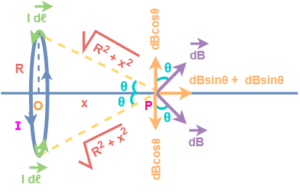
The effective magnetic field due to any current element of the loop will be-

 

 

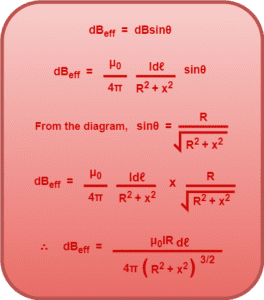
The net magnetic field at point P due to all such current elements of the loop is given by-

 

 

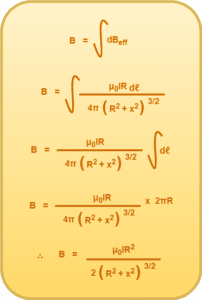
Thus, the net magnetic field at the axial point P of a circular current carrying loop is given by-

 

 

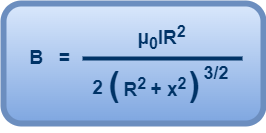
If instead of a single loop, there is a coil of N turns all wound one over another, then-

 

 

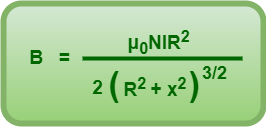
Special Cases-

 

Case-01: At the center of the loop-

 

If an axial point lying at the center of the loop, we put x = 0 in the above formula, then we have-

 

 

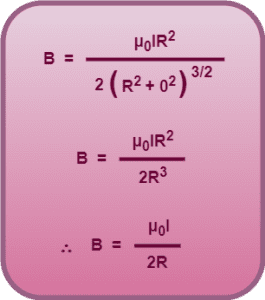
It is important to note that this is the maximum value of the magnetic field.

 

Case-02: At the axial points lying far away from the loop-

 

For all such points, we have x >> R. So,

R² + x² ≈ x²

Using in the above formula, we have-

 

 

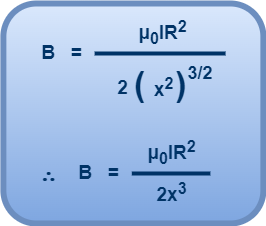
Case-03: At the axial points at a distance equal to radius of the loop-

 

For such points, we put x = R in the above formula, then we have-

 

 

Graph Showing Variation of Magnetic Field-

 

The following graph shows the variation of magnetic field along the axis of a circular current carrying loop with distance from its center-

 

 

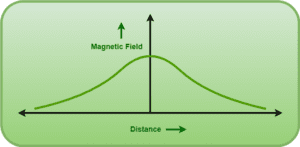
• The value of magnetic field is maximum at the center.
• It then decreases as we go away from the center on either side of the loop.
• The value of magnetic field is minimum when x = ± ∞.

 

Very Important Note The direction of magnetic field is same on either side of the loop.

 

MCQs Quiz-

MCQs Quiz

on Magnetic Field On Axis of Circular Current Loop

 

Worksheet-

Worksheet

on Magnetic Field On Axis of Circular Current Loop

 

Next Article-

Current Carrying Circular Loop As A Magnetic Dipole

 

Get more notes & other study material of the Chapter Moving Charges & Magnetism.