Coulomb’s Law-

 

Before you go through this article, make sure that you have gone through the previous article on Coulomb’s Law.

 

We have learnt-

• Coulomb’s Law helps to calculate the magnitude of electric force between the two stationary point charges.
• By Coulomb’s law, the the magnitude of electric force between the two charges is calculated as-

 

 

In this article, we will learn about Coulomb’s Law in Vector Form.

 

Coulomb’s Law In Vector Form-

 

Let q₁ and q₂ be two like charges placed at points A and B respectively in vacuum as shown-

 

 

Using triangle law of vector addition, we have-

 

 

The coulomb’s force F₂₁ acting on charge q₂ due to charge q₁ is given by-

 

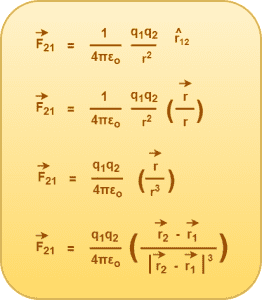
Equation-01

 

The coulomb’s force F₁₂ acting on charge q₁ due to charge q₂ is given by-

 

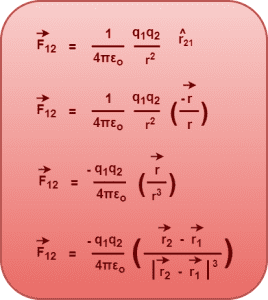
Equation-02

 

Equations-01 and 02 gives Coulomb’s law in vector form.

 

Importance of Vector Form-

 

The vector form of Coulomb’s law gives the following additional information-

 

1. F₁₂ = – F₂₁. This means that the two charges exert equal and opposite forces. So, Coulombian forces obey Newton’s third law of motion.

2. As the Coulombian forces, F₁₂ and F₂₁ act along the line joining the centers of two charges, so they are central forces.

 

NOTE If we put q1 and q2 with proper signs in equations-01 and 02, then the direction of forces automatically gets adjusted.

 

Read the next article on-

Electric Force | Definition | Properties

 

Get more notes & other study material of the Chapter Electric Charges & Field.