Gauss’s Law Proof | Prove Gauss’s Law

Gauss’s Law-

 

Before you go through this article, make sure that you have gone through the previous article on Gauss’s Law.

 

We have learnt-

• Gauss’s Law helps to find the total electric flux through any closed surface.
• It states that the total electric flux through any closed surface is 1/ε_o times the net charge enclosed within it.

 

In this article, we will discuss the proof of Gauss’s law.

 

Gauss’s Law Proof-

 

Consider an isolated point charge +Q placed at a point O. We draw a spherical Gaussian surface of radius R with O as the center and calculate the electric flux from it.

 

 

The electric field intensity due to charge +Q at every point on the Gaussian surface is given by-

 

 

Also Read- Electric Field Due To A Point Charge

 

The electric flux over the entire closed Gaussian surface is given by-

 

 

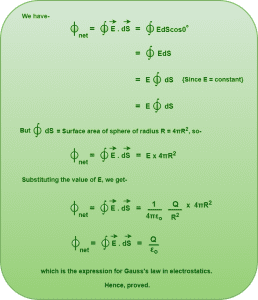
This proves Gauss’s law in electrostatics.

 

Read the next article on-

Deducing Coulomb’s Law From Gauss’s Law

 

Get more notes & other study material of the Chapter Electric Charges & Field.