Combination of Resistors-

 

Sometimes, a number of resistors are connected in a circuit in order to get a desired value of current in the circuit.

 

Resistors may be combined in a circuit in the following ways-

 

 

1. Series Combination
2. Parallel Combination
3. Mixed Combination

 

In this article, we will learn about series combination of resistors.

 

Series Combination of Resistors-

 

Two or more resistors are said to be connected in series combination if- they are connected end to end with each other the same current flows through each one of them in succession when connected to a cell or a battery

 

Expression For Equivalent Resistance-

 

Consider-

• Two resistors of resistances R₁ and R₂ are connected in series combination across a battery.
• The battery maintains a potential difference V across the combination.

 

 

Using Kirchoff’s Voltage Law in the above circuit, we have-

 

-IR₁ – IR₂ + V = 0

-I (R₁ + R₂) + V = 0

V = I(R₁ + R₂)       ………..(1)

 

Also Read- How to Apply Kirchhoff’s Laws?

 

Now, we wish to replace the above series combination of resistors with a single equivalent resistor of resistance R_eq.

 

An equivalent resistor is one which draws the same current from the battery as drawn by the individual resistors together.

 

Replacing the above series combination of resistors with an equivalent resistor, we have-

 

 

Using Kirchoff’s Voltage Law in the above circuit, we have-

 

-IR_eq + V = 0

V = IR_eq        ………..(2)

 

On comparing equations (1) and (2), we get-

 

 

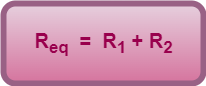
This is the required expression for equivalent resistance of a series combination of two resistors.

The formula can be extended for n resistors in the same way.

 

In series combination, the equivalent resistance is equal to the sum of the individual resistances.

 

Important Notes-

 

Note-01:

 

In series combination,

• The current flowing through each resistor is same but potential difference divides among the individual resistors.
• The potential difference across the combination is equal to the sum of potential difference across the individual resistors.

 

Note-02:

 

The equivalent resistance of a series combination of resistors is equal to the sum of their individual resistances.

 

 

Note-03:

 

If n resistors each of resistance R are combined in series combination, then their equivalent resistance is given by-

R_eq = R + R + ……. n times

Thus,

 

Note-04:

 

In series combination, the equivalent resistance is larger than the largest individual resistance.

 

Note-05:

 

The largest value of equivalent resistance that can be obtained with the given resistances is by combining them in series combination.

 

Test Your Concepts-

Quiz on Series Combination of Resistors

 

Next Article-

Parallel Combination of Resistors

 

Get more notes & other study material of the Chapter Current Electricity.